Physics 2212, Lab 11: Induction

Eric Murray, Fall 2006

Question these experiments will enable you to answer: How does the peak induced emf in the secondary coil of a transformer depend on the peak current supplied to the primary coil, the frequency of the current supplied to the primary, and the length of the primary within the secondary?

Features: An automated system will supply and record a sinusoidally varying current to the primary coil of an air-core transformer. The system will record the emf induced in the secondary coil. Sine functions will be fit to both the current and emf data so the amplitudes (peak values) may be found.

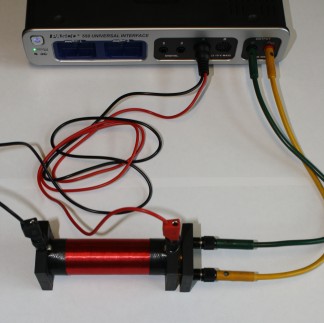Preliminaries: Current is supplied to the primary (narrow, inner) coil by the output sockets. Potential is measured across the secondary (wide, outer) coil by a voltage sensor in analog channel A. (The supplied current will be measured by the PASCO interface internally.) Open the data template. You'll find graphs for the current through the primary and the potential across the secondary as a function of time. The signal generator is set to provide a potential difference that varies with time as a 5.0 kHz sine wave having a 0.050 V amplitude. Data will be collected for 0.004 s after Monitor is clicked. Nothing need be calibrated.

Experiment 1 — Current Dependance: Click Start. Fit sine functions to the primary current and the secondary potential graphs. Record the amplitudes. Repeat for a total of ten primary potential amplitudes, in 0.050 V increments. Find the slope of the _s0 vs. I_p0 graph, along with its uncertainty and correlation coefficient. You may find Excel to be helpful. Note that the square of the correlation coefficient is roughly the fraction of the change in _s0 that can be attributed to changes in I_p0.

Experiment 2 — Frequency Dependance: Set the Signal Generator control to a 0.10 V, 1.0 kHz sine wave. Once again, click Start and fit sine functions to the primary current and the secondary potential graphs. Record the amplitudes. Repeat for a total of ten primary potential frequencies, in 1.0 kHz increments. Since the current does not remain constant, find the slope of the _s0 / I_p0 vs. f graph, along with its uncertainty and correlation coefficient. Once again, you may find Excel to be helpful.

Important! Pay close attention to the fit sine curve that is displayed on your data. Particularly at higher frequencies, the curve fit routine may find a sine function that does not really fit your data. Make sure that the period of the fit to the induced emf data matches the period of the fit to the supplied current data. If they do not match, try fitting a sine series curve to your induced emf data. This will fit the sum of two or more sine functions to your data, and display information about each one. Choose the one with a period that matches the period of the fit to the supplied current data. On rare occasions, none of the sine functions will have a suitable period. In that case, try recording a new set of data for that frequency.

Experiment 3 — Length Dependance: Set the Signal Generator control to a 0.20 V, 5.0 kHz sine wave. With the primary coil fully within the secondary, click Start and fit sine functions to the primary current and the secondary potential graphs. Record the amplitudes. Repeat for a total of ten measurements, withdrawing the primary 1.0 cm each time. Although the current shouldn't vary as much as it did when the frequency dependance was investigated, it may still vary a little. Therefore, you should graph _s0 / I_p0 vs. various functions of length (1 / l², 1 / l, l, and l² are suggested). Report which function has a linear relationship, along with the uncertainty and correlation coefficient for that function. Excel may still be helpful.

Summary: Review your worksheet. Think about the goals of these experiments, your results, and the expectations from theory while writing your discussion.